Hi, not having a good time right now. Been Ill and am very deep in car repair that have gone wrong in many ways at every step. Stress is an understatement
So, I’m trying to wrap my head around this but sadly I missed a lot of maths at school and I get as far as parentheses goes first and then split.
If you can’t explain, but can do the sums. Please change 600 to 380.
Thanks in advance
Hi LocalYocal. Sorry you’re having a hard time.
Problem looks a bit technical, but as far as the calculation goes, are you asking what would be the effect on the final number (0.27, the mach) if the (airflow) 600 was changed to 380?
If so, I think as the 600 is a multiplicative term (and so is the resulting 293) this would simply reduce the 0.27 proportionately ie times it by 380/600.
Sorry if this isn’t what you are asking!
ED
Thanks bud. Last week has been…fun. Appreciate the thought.
As for the puzzle, I’d like to think you approach would work, but this matters and I want to get it right because I don’t want to have to touch it again.
Turbocharging is a fantastic subject. A pump with a couple of moving parts. But oh boy is it complicated. Rocket science, literally. Keeps me occupied, what with the world and mother too.
Hi @LocalYokel
I agree with Evvy. The main formula is
velocity = airflow / area
If you keep the area the same and decrease the airflow from 600 to 380 it reduces the velocity proportionally.
The complex multiplication is made up of 2 parts. One doing the main calc, and the other doing some faffing around with units to convert inches to feet and minutes to seconds, because the author wants the end result in feet/second not inches / minute.
That means you can just forget about the second multiplication term basically. It is a factor of 2.4 that you apply to get the right units. If you’re measuring your pipe diameter in inches, and your airflow in CFM as the author is doing then a simplified version of the calc is:
Airflow (CFM)
__________________________ x 2.4
π x (1/2 x pipe diameter)^2
So in the example:
600 ÷ (3.1415 x 1.25 x 1.25) x 2.4 = 293 and change
To go from 600 to 380 and keeping the rest the same, you get:
380 ÷ (3.1415 x 1.25 x 1.25) x 2.4 = 185.8
Which is exactly the same as @Evvy_dense
380÷600 x 293 = 185.6
Hope that was helpful
Thanks for taking the time to explain it, it’s much appreciated.
For the record. 0.17313. 45mm pipe will do nicely.
Glad.
For the record though, you’ve worried me by mentioning a 45mm pipe! Measurements really should be in feet and inches to make the formula above work - especially with the 2.4 fudge factor! 2.5 inches should be 63.5mm pipe.
Hope I’ve not made things worse now!
You haven’t made things worse. It’s partially my fault. The answer after dividing by 1100 was 0.17313. This is a couple of fag papers away from 1.75 in. 1.75 in=45mm.
The other part of the fault lies in bloody plumbing. Couplers, fittings and whatnots come in all sizes and often with a different sized nut to top it off (which is why plumbers use adjustable wrenches and spanners). Yet hydraulic connectors are uniform…
I was given some 63mm piping and tested it. More power at top end, but drivability and response disappeared so I put the factory kit back on (for now).
Thanks again bud
Hi companero
glad, you’re happy but im not 100% following along. Probably me that’s being think here (not the first time).
380 ÷ (3.1415 x 1.25 x 1.25) x 2.4 = 185.8
185.8/1100 = 0.1689
As I understand the original, this is Mach 0.17 (a velocity). I’m not sure about what that means for 1.75" pipe… As i said, I’m no doubt missing an important point here, but as you were keen on grokking the whole thing, I thought I’d raise this again.
Cheers
EDIT
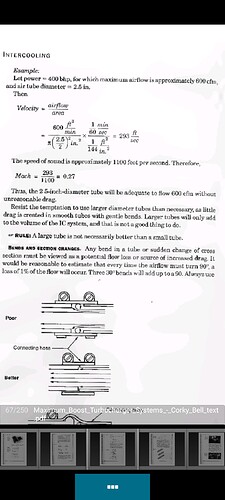
looking again at the book snippet you posted. The Mach number seems important as it directly relates to the drag in the pipe. Higher Mach number will give higher drag. The 2 diagrams at the bottom seem to be talking about that too - one shows turbulent flow inside the pipe (presumably the Mach number was too high) and the second shows laminar flow. It feels to me like the author is saying that as the Mach number is low (0.27) the 2.5 inch pipe is fine to handle airflow of 600 cubic feet / minute.
If you reduce the airflow from 600 → 380 but want to keep the Mach number about the same, we can reduce the pipe diameter, but it’s not so straightforward. We have to solve this equation for the pipe diameter:
Airflow (CFM)
__________________________ x 2.4 = 293 (=Mach 0.27)
π x (1/2 x pipe diameter)^2
We know the airflow is 380, so in other words what pipe diameter solves this equation?:
380
_____________________ x 2.4 = 293
π x (1/2 x pipe diameter)^2
a bit of messing about and we get:
380
______ x 2.4 = (1/2 x pipe diameter)^2
π x 293
or finally that the pipe diameter for an airflow of 380 CFM and a Mach number of 0.27 is:
pipe diameter = 2 x square root ( 2.4 x 380 ÷ (π x 293) )
pipe diameter = 2 x 0.995 = 1.99 inches.
so a 2 inch pipe would give you the same drag for an airflow of 380 CFM as a 2.5 inch pipe would for an airflow of 600 CFM.
I might be way off base here, in which case I’ll come back and delete all the unnecessary crap. But thought I’d post it up in case it’s still helpful
Ahoy.
Above Mach 0.4 or approximately 450ft p/s drag starts to become a serious issue. More modern suspicion lies at around 400ft p/s. So the trick is to use the smallest possible diameter that keeps flow below 400ft.
Whilst the equation gives an answer of 0.1698, we have to move the decimal place giving 1.698in. Which is 42.9mm in modern money. Smack between 41 and 45mm choice. I’ll go for the larger one.
Edit
LOL. Now I’m back to my Original suspicion that 2mm is perfect.
